ASTR 511 (O'Connell) Lecture Notes

TELESCOPE OPTICAL DESIGN

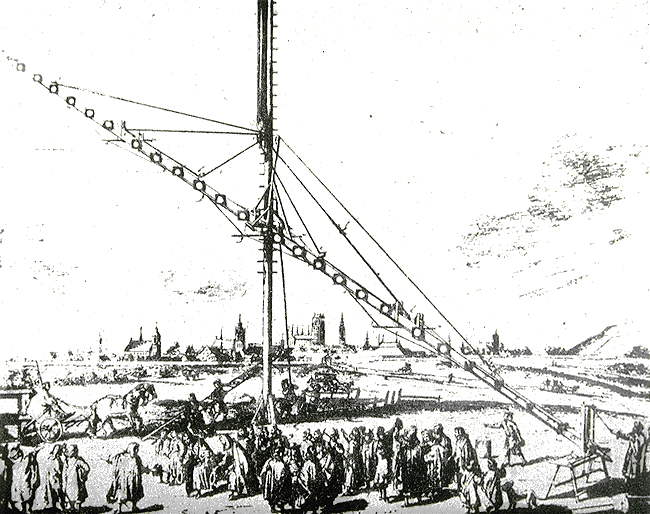
Hevelius 140-foot Telescope (ca. 1675)

Telescope optical design is covered in detail in ASTR 512, so we give only a flavor of what is involved here.

A. Overview

The usual goal of telescope optics design is to bring all light rays, arriving at the primary element from a large range of field angles, to a sharp focus in a flat focal plane. The image should be a faithful one-to-one reproduction of the angular distribution of light in the source with minimal geometric distortion and image blur. The image representation should be independent of the wavelength of the light rays (i.e. achromatic).

But it is not possible to accomplish this. One can never build a perfect optical device. Any realized design is always a compromise because it is not possible to control for all optical aberrations at will.

Therefore, modern telescope design is essentially a process of successive approximations in aberration control in 3 dimensions. Fortunately, a number of highly capable software packages are now available to mitigate the pain of doing this. In the case of large mirrors or multiple-mirror designs, continual mechanical surface correction and alignment devices must be included.

The picture at the top of this page shows how early telescope builders tried to control for two major aberrations in crude lenses: (1) chromatic aberration and (2) spherical aberration. The effects of both of these aberrations diminish with focal length....hence the extraordinarily long focal length of Hevelius' telescope.

The modern solution to chromatic aberration (the fact that the focal point depends on the wavelength) in refractive optics is to use mirrors rather than lenses, and most large telescopes employ mirrors as their primary optics. However, most optical instruments must also include refractive elements in order to achieve wavelength selection or wide-field images, so chromatic aberration is still almost always an issue.

The modern solution to spherical aberration (the fact that parallel light rays striking the surface of a sphere do not come to a single focus) is to use non-spherical optics -- e.g. paraboloids and hyperboloids. Rays parallel to the axis of a parabolic reflector come to a perfect focus. Unfortunately, off-axis rays do not, and this leads to a comet-shaped aberration known as coma, which becomes worse with field angle.

Coma can be reduced by using a refractive corrector near the focal plane of a parabolic primary.
A widely-used alternative is the Ritchey-Chretien design, a Cassegrain system that employs two hyperbolic mirrors yielding zero first-order coma and spherical aberration. RC's are affected, however, by third-order coma, astigmatism, and field curvature (at larger field angles).
Another famous alternative for control of spherical aberration over a wide field with a spherical primary is to use a special full-aperture refractive correcting plate called a Schmidt corrector. The Schmidt sky-survey telescopes employ these.

B. Sample Ray Traces

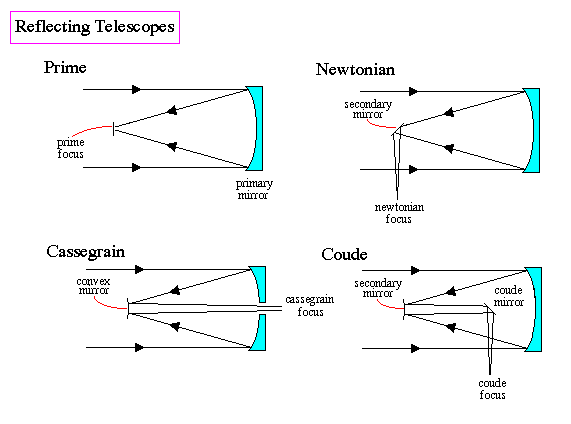
Click below for illustrations of ray traces for spherical and parabolic reflectors. These show light paths for parallel incoming rays in a plane containing the symmetry axis of the mirror. They are for fast optical systems (small f/ ratios, where f/ = Focal Length/Diameter), so that aberrations are larger than for typical designs and can be seen in the diagrams. You can see that, except for the case of a parabola and paraxial rays, it is not possible to find a good focal point anywhere in these systems.

STANDARD TELESCOPE OPTICAL CONFIGURATIONS

TYPE	PRIMARY MIRROR	SECONDARY MIRROR	COMMENTS
PRIME FOCUS	Parabola		Focus inside telescope. Add refractive corrector for wide field.
NEWTONIAN	Parabola	Flat	Focus at side/top of telescope.
CASSEGRAIN	Parabola	Hyperbola (convex)	Focus below primary.
GREGORIAN	Parabola	Ellipse (concave)	Primary mirror focus before secondary. Focus below primary.
RITCHEY- CHRETIEN	Hyperbola	Hyperbola (convex)	Focus below primary. Zero coma & spherical aberration.
COUDE	Various	Various	Tertiary flat directs light to fixed focus below polar axis.
NAYSMITH	Various	Various	(Alt-Az): Tertiary flat directs light to focus outside altitude axis.
SCHMIDT	Spherical		Catadioptric: Uses refractive corrector to provide wide field without spherical aberration. Focus inside telescope body.

Back to Lecture 16

Last modified November 2020 by rwo