ASTR 1210 (O'Connell) Study Guide
4. MOTIONS IN THE SKY

Star trails over the Himalayas in a
2-hour exposure
(Anton Jankovoy).
A. Motions of Bright Objects
The table below lists celestial motions which are easily detectable by someone on the Earth without telescopes.| OBJECT | PERIOD | MOTION |
|---|---|---|
| ALL | Daily ("diurnal") | Rotation Westward |
| SUN | Annual (365 days) | (a) 1 degree/day Eastward* |
| (b) North/South* | ||
| MOON** | Monthly (29 days) | (a) Eastward, N/S* |
| (b) Phase change | ||
| PLANETS (5)** | Months-Years | Generally Eastward, but with Westward loops* |
-
*Except for the diurnal motion, motions are measured with respect to the stars.
The diurnal motion is measured with respect to the local horizon.
**The Moon and the planets move on paths near to, but not identical with, the annual path
of the Sun seen against the stars (the "ecliptic")
-
People are familiar with the motion of the Sun during the day. That
is the most obvious manifestation of the "diurnal motion" of the whole
sky. But the other motions listed are less conspicuous and so slow
that if you aren't a practicing amateur astronomer, you probably
aren't aware of them.
If you're patient for about 20 minutes, you can easily detect the
diurnal wheeling rotation of the whole night sky by comparing
the position of the stars to a fixed foreground object (e.g. a tree).
If you have a digital camera capable of taking exposures longer than a
few minutes, you can readily take images showing star trails,
like the one at the top of the page.
The best way to visualize celestial motions is in a planetarium or
with a good computer sky simulation program. We will use
the Starry Night
simulator in class.
But a faster way to experience the diurnal motion is to view one of
the many excellent time-lapse
videos now available on the Web.
B. Explanation of Motions
In the rest of this guide, we explain these phenomena from a modern scientific perspective. It took many centuries for astronomers to arrive at the correct interpretation described here. Ancient Greek astronomers understood much of this after several hundred years of work, but the knowledge was lost and only rediscovered during the Renaissance, 1300 years later. The key to complete understanding of celestial motions was introduced by the Greeks: mathematics.- The Greeks built mathematical models of the sky based on plane and spherical geometry which they had developed. These reduce a bewildering array of complex phenomena to a much simpler set of mathematical concepts.
- Although it is fashionable today to criticize "reductionism" in science, there would, in fact, have been very little progress in understanding nature (or in converting that understanding to useful technologies) without the tremendous simplifying insights of reductionism.
- Intrinsic motions of the objects themselves with respect to one another
- The motion of the observer, or the platform on which
he/she is standing---in this case, the Earth
-
Because the motions of objects in the sky can be induced by movement
of the observer, we call them apparent motions.
- THE EARTH WE LIVE ON IS A ROUND, TILTED, SPINNING, MOVING PLATFORM.
- Although it is simple to state, it is very difficult for most people to visualize this situation. You instinctively feel yourself to be "upright" and at rest on a flat, stationary Earth, but your instinct here is seriously misleading. This is one of the main reasons it took humans nearly 2000 years to fully accept this explanation, which became the first major discovery of the scientific age (by Copernicus ca. 1540, see Study Guide 6).
- The motions of the Moon and the planets are described on the Lunar Motions page and in Study Guide 5, respectively.
C. Effects of Earth's Shape and Spin
- Earth is a sphere, with a diameter of 7900 miles.
- Half of Earth is always in sunlight; half is always in shadow. We experience night on the shadow side (away from the Sun).
- Daylight = sunlight scattered by the Earth's atmosphere
whenever the Sun is above the horizon. The daylight glare overwhelms
the light of the planets and stars, so we cannot see them (though they
are, of course, always there).
- The glare rapidly declines as the Sun
dips below the horizon ("dusk"). "Civil twilight," the point at which
sunlight is just barely perceptible, occurs when the Sun is 6 degrees
below the horizon.
- Earth spins on its axis with respect to the stars once in
23 hours, 56 minutes (one "sidereal day").
-
This causes the
apparent diurnal rotation of the whole sky. (The universe is not
moving around the Earth once per day.)
If the Earth were assumed not to be spinning, then the
diurnal movement of the sky would necessarily imply that it was at
the center of the universe. This was the first assumption
discarded by Copernicus on his way to
rejection of the ancient "geocentric" cosmologies.
- Earth's spin is counterclockwise (eastward) as seen from above
Earth's N pole; this means that the apparent rotation of the sky seen
from Earth's surface is westward.
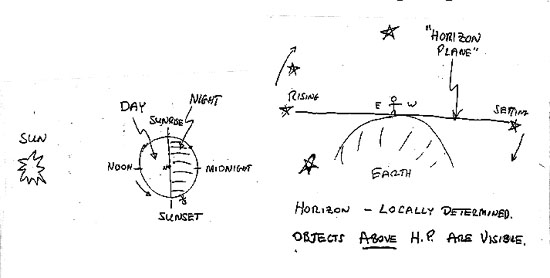
- The left-hand panel of diagram above shows Earth viewed from above its
North Pole. The Earth rotates counterclockwise in this diagram,
carrying observers with it.
- The positions where observers on the equator are experiencing sunrise, noon, sunset, and midnight are marked. Mean local times of day corresponding to these points are 6 AM, 12 noon, 6 PM, and 12 midnight, respectively.
- The right hand panel shows the local "horizon plane,"
which is the plane "tangent" to Earth at your location. You can see
(in principle) objects above the plane but not below it.
- Note that half of the entire "celestial sphere" (see Study Guide 3) is always above your horizon plane. However, because the Earth is spherical, the horizon plane (and the visible hemisphere) is different at each location on the Earth's surface.
- The horizon plane sweeps across the sky as Earth spins. Astronomical objects appear to move in the opposite direction (shown by the arrows in the figure). Objects rise over the eastern horizon and set toward the western horizon.
- The left-hand panel of diagram above shows Earth viewed from above its
North Pole. The Earth rotates counterclockwise in this diagram,
carrying observers with it.
- By combining the concept of the horizon plane with the definition of local time in the left-hand panel, you can visualize what parts of the sky are observable at any given time at a given location on the Earth.
D. Effects of Earth's Motion in Orbit
- Earth is a planet moving in orbit around the Sun
- Its orbit is nearly circular (the distance to the Sun varies only 3.4%), with a mean radius of 150,000,000 km or 93,000,000 miles. The mean radius is defined to be the Astronomical Unit (AU).
- Its orbit lies in a plane called the "ecliptic" plane. Seen face-on, the orbit is technically an ellipse but deviates only slightly from a circle. Seen edge-on, the orbit is a thin line.
- Earth orbits the Sun in 365.25 days (one year) moving at ~66,000 mph. Its motion is counterclockwise as seen from above the north pole.
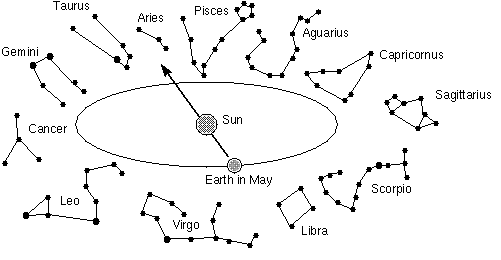

- Stars visible at night are those "opposite" the Sun. See the two
figures above. The night side of Earth is that opposite the Sun. So,
in May, the constellation Scorpio will be prominent in the night sky,
while in November, it lies in the direction of the Sun and therefore
is not visible because of the daytime atmospheric glare.
- Warning! the drawings in this guide,
and most others you will see in this course either in the text or in
class, are grossly distorted and not to scale! Don't take them
literally. In contrast to the drawing above of the Earth's orbit, the
real orbit is 100 times the diameter of the Sun; the Earth itself is
100 times smaller than the Sun; the stars are vastly distant from the
Earth's orbit; and the stars in a given constellation are not
necessarily near one another in space.
- Obviously, no one could produce or sensibly view a figure like this drawn to actual scale.
- We are viewing Earth's orbit here from an inclination such that it looks highly elliptical, whereas it is nearly circular seen face-on.
- Warning! the drawings in this guide,
and most others you will see in this course either in the text or in
class, are grossly distorted and not to scale! Don't take them
literally. In contrast to the drawing above of the Earth's orbit, the
real orbit is 100 times the diameter of the Sun; the Earth itself is
100 times smaller than the Sun; the stars are vastly distant from the
Earth's orbit; and the stars in a given constellation are not
necessarily near one another in space.
- The Earth's motion around the Sun is counterclockwise in
the drawings above (drawn from a viewpoint that is north of the Earth's
orbital plane), so that in August, the Earth's position would be
nearest the drawing of Capricornus in the diagram.
- Earth's orbital motion produces an apparent eastward "reflex motion" of the Sun in the sky against the stellar reference frame as seen from the Earth.
- From the drawings, we find that the Sun in November would be seen in projection in the direction of Libra, while in December, 30 days later, the Earth has moved such that the Sun is seen in projection against Scorpio, which is approximately 30 degrees east of Libra. Therefore, the average "reflex motion" of the Sun with respect to the stars as seen from Earth is 1 degree per day eastward.
- The ecliptic path:
- The Sun's annual path seen from the moving Earth against the stars is called the "ecliptic path." This is simply the projection of the ecliptic plane on the celestial sphere.
- The "Zodiac" is the set of constellations through which the ecliptic path passes. Those are the only constellations which have been illustrated in the drawings above. Of course, there are 76 other constellations not shown.
- The only astronomical significance of the 12 Zodiacal constellations, therefore, is accidental: they happen to lie on the projection of the plane of the Earth's orbit. They are not the largest, brightest, or most prominent constellations (in fact a number are quite inconspicuous).
- Practice exam question: which Zodiacal constellation will be best seen at midnight in August?
- "Solar" vs. "sidereal" days: The daily motion of the Earth in its
orbit means that the Earth must spin a little more than once
on its axis to bring the Sun back to the point of local "noon" (i.e.
halfway between rise and set). The extra amount is 4 minutes, on
average.
- The effect of Earth's motion during one sidereal day is illustrated here.
- This accounts for the difference between the sidereal spin
period of the Earth (23 hours, 56 min) and the average
elapsed time between successive noons (24 hours, the
"solar day"). Our ordinary clocks, of course, are set such that the
time between successive noons is defined to be 24 hours. But
the stars come back to the same position in the sky as seen from
Earth 4 minutes earlier each day. This means, for instance,
that the stars you see at 11 PM in a given month have the same
location with respect to the horizon as they did at 9 PM one month
earlier or 7 PM two months earlier.
E. Tilt of Earth's Axis
- The polar rotation axis of the Earth is not perpendicular to
its orbital plane. It is tilted by 23.5 degrees from the vertical,
or, equivalently, it is tilted 66.5 degrees out of the plane. See figure
below (again, exaggerated for clarity):
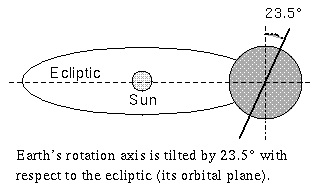
- The rotation axis is fixed in direction with respect to the
stars, not with respect to the Sun. As Earth
orbits the Sun, the axis continues to point in the same direction
in 3D space. This implies that the north pole is sometimes
"tilted toward" the Sun, sometimes "tilted away." In the illustration
above, the North Pole is shown at its maximum tilt away from the Sun
(assuming the North Pole is at the top).
-
The fixed axis direction of the Earth during a year is illustrated
in the drawing in Section F below.
- This, in turn, implies that the Sun, as viewed from the Earth,
will appear to move north and south of the celestial equator
through the year by a maximum of + or - 23.5 degrees. In the drawing
above the Earth is situated such that the Sun will be seen at its most
southerly position during the year.
-
The total amplitude of the Sun's N/S swing across the celestial
equator is 2 x 23.5 = 47 degrees.
- Equivalently, the ecliptic path is inclined 23.5
degrees to the celestial equator. The following diagram shows
the ecliptic and the equator on the celestial sphere (see Guide 3 for the definition of the celestial
sphere).
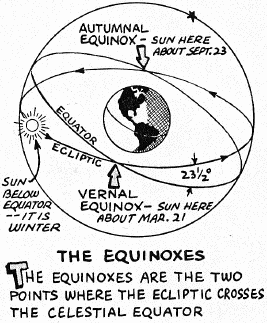
- The ecliptic crosses the celestial equator at two points
called equinoxes.
- When the Sun is at an equinox, night and day are each 12 hours long at all latitudes. The "Vernal Equinox" in the northern hemisphere occurs around March 21, while the "Autumnal Equinox" occurs around September 21.
- The Sun is at its greatest distance from the equator (23.5 degrees) at the solstices ("sun stationary"), which are approximately June 21 and Dec 21. At these times, one hemisphere experiences its longest day, the other its shortest.
- The diagram below shows the position of the Sun on the celestial sphere with respect to the equator plotted against date. The longest and shortest days in the northern hemisphere occur at the June and December solstices, respectively. (The labeling in the diagram is for the northern hemisphere; the seasons are reversed in the southern hemisphere.)

F. Astronomical Effects on the Weather
- The Sun's north/south distance from the celestial equator
determines:
- The number of hours of daylight at a given latitude and
- The angle at which sunlight strikes Earth's surface at a given latitude. More energy is incident per unit area per second on the surface the closer are the rays to perpendicular to the surface.
- The Sun's angular distance from the celestial equator thus determines insolation, or the amount of solar energy deposited on a given area of the Earth's surface during 24 hours at a given latitude.
- This differential heating is responsible for the seasons. The "official" beginning dates of spring, summer, autumn, and winter correspond to the Vernal Equinox, Summer Solstice, Autumnal Equinox, and Winter Solstice, respectively.
-
The diagram below shows how the hours of daylight (represented by
the length of the white lines on the illuminated half of the Earth)
and the solar angle of incidence at different latitudes correlate with
the date. A close-up of the situation is shown here.

- The change in the geographic shadow distribution caused by the
tilt is quite dramatic (even though the shadow always covers exactly
one half of the Earth's surface). Below are two images of the way the
shadow is distributed at two different times of year. The Earth's
surface moves eastward (toward the right in the diagrams) through the
shadow as the Earth rotates. You can immediately tell from the image
which latitudes are receiving more sunlight in a 24 hour period.
Click on the thumbnails for an expanded view.

2 PM EDT August 1 2 PM EST December 1 -
 Here
is a nice timelapse video compilation of images from space of the
Earth's surface at the Autumnal Equinox in 2013.
Here
is a nice timelapse video compilation of images from space of the
Earth's surface at the Autumnal Equinox in 2013.
- Therefore, the seasons are caused by the tilt of the Earth's
pole to its orbital plane, not its distance from the Sun.
-
If the seasons were a distance effect, winter, for instance, would
occur simultaneously in both the southern and northern
hemispheres. But instead, the seasons differ by 6 months in the two
hemispheres.
There is a small annual change in the distance of
the Earth from the Sun caused by the finite ellipticity of Earth's
orbit. This amounts to only + or - 0.017 AU. The change in distance
has a real, but small, effect on the seasons. The Earth is nearest
the Sun in January, one of the coldest months in the northern
hemisphere. The seasonal changes therefore are milder in the northern
hemisphere and larger in the southern hemisphere than they would have
been if Earth's orbit were perfectly circular.
- Local Weather Extremes:
-
The change in insolation at the mid-latitudes (like
Charlottesville's) would not by itself cause the extremes of weather
we experience here (e.g. frigid winter temperatures). Instead, it is
mixing by wind currents of air masses from different
latitudes that produces the extremes. In the case of winter, the
north polar regions experience perpetual night starting in September
and lasting until March. The resultant drastic atmospheric cooling
creates the icy air masses that can produce very cold weather in the US
if they move over us.
- The Milankovitch Effect
-
The polar tilt and the properties of the Earth's orbit change
slightly with time over long periods (10's of thousands of years)
because of the gravitational effects of the other bodies in the solar
system. Small changes in the polar tilt, the size and shape of
Earth's orbit, or the timing of closest approach to the Sun can have
cumulative effects on heating of the atmosphere.
One of the important effects is
precession, discussed on the
Lunar Motions page, which changes
the timing of the solstices with respect to the Earth's distance
from the Sun.
There is good geophysical evidence for a correlation
between such astronomical changes and
major changes in climate over long time periods, particularly
in the form of ice
ages. This is known as the "Milankovitch Effect."
Astronomical and atmospheric effects on climate are discussed further
in Study Guide 19.
- The Milankovitch Effect
G. Effects of Intrinsic Lunar Motions
Reading for this lecture:
-
Bennett textbook: Ch. 2.1, 2.2
Study Guide 4
Lunar Motions and Their Consequences
Puzzlah preparation exercise. (Work alone or in a small group.)
- How wide is the Sun in degrees?
- [Optional] Repeat this exercise for the star cluster the Pleiades (in the constellation Taurus) viewed on a clear night. Which is larger, the Sun or the Pleiades?
- [Optional] If the whole sky (both hemispheres) covers 41,000 square degrees, how many times over could you fit the Sun's apparent disk into the whole sky?
- Measure the angular diameter of the Sun as follows. You
can do this on any day (clear or partly cloudy) when you can see the
disk of the Sun. Don't look directly at the sun. Instead put your
hand (palm out & fingers together) in front of your eyes at arm's
length. Close one eye. Then, carefully fold down fingers, keeping the
Sun's light covered until you can't remove any more fingers without
letting sunlight pass. Remember that your index finger will subtend
about 1 degree in width when held at arm's length.
-
Bennett textbook: CH 2.3 (lunar phases, eclipses), 3.1 (ancient astronomy)
Study Guide 5
Aztec Calendar Stone
Lunar Motions and Their Consequences
Optional reference on Mayan astronomy: Skywatchers of
Ancient Mexico by Anthony F. Aveni (Univ. of Texas Press,
1980/97).
Puzzlah Preparation Questions
Web links:
-
Spectacular star trail
photos by L. Harrison
Thanks to the capabilities of modern digital cameras for taking
hundreds of images in sequence, there are now scores of beautiful
time-lapse videos of the night sky, the Earth, and views from
the International Space Station made by both professional and amateur
astronomers. Links to some good video sites are given on
the ASTR 1210 Web Links Page.
Interactive
Earth & Moon viewer (includes software & add'l links)
JPL Tutorial on Earth motions and astronomical reference systems
Ice Ages (Wikipedia)
Lecture on the Milankovitch Effect (J. Frogel, OSU)
Milankovitch Cycles (Wikipedia)
 Previous Guide
Previous Guide
|
 Guide Index
Guide Index
|
 Next Guide
Next Guide
|