ASTR 1210 (O'Connell) Supplement
LUNAR MOTIONS AND THEIR CONSEQUENCES

A. Lunar Phases
Unlike the planets and stars, the Moon is perceptibly extended on the sky to the unaided eye. Throughout a given month, the Moon exhibits drastic changes in apparent shape, from crescent to round and back. The shapes are called phases of the
Moon. Overall lunar brightness changes in proportion to
the bright area. .
The shapes are called phases of the
Moon. Overall lunar brightness changes in proportion to
the bright area. .
- A montage of photographs of the lunar phases during a month is shown at the right. Click for an enlargement.
- Other than the apparent daily and annual motions of the Sun, the lunar phases are the most dramatic of the cycles visible in the sky. The Moon repeats its phases after a period of 29.5 days.
- Lunar phases were especially important before the invention of artificial lighting, because the Moon's brightness directly affected people's ability to move around at night. Phases near "full" Moon produce very considerable illumination of the Earth's surface. That's a benefit for normal human activity. But in compensation, scattered Moonlight in the atmosphere at those times is sufficient to conceal all but the brightest planets and stars from easy visibility.
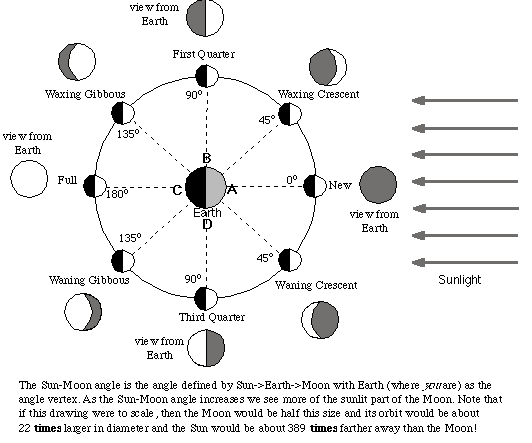
- The key clue is that the phase of the Moon correlates directly with its angular distance from the Sun in the sky. The Moon is "rounder" the farther from the Sun it is in angular distance. See this illustration, which shows the phases and location of the Moon at sunset during the first two weeks of a lunar cycle. The Sun is always at the western horizon -- i.e. Sunset -- at the times shown.
- Having invented solid geometry, the Greeks realized that this behavior implies that the Moon is a sphere, in orbit around the Earth, half of which is always illuminated by the Sun. It is not self luminous and shines only by reflected light.
- The fraction of the sunlit hemisphere which we can see from Earth at any time determines the lunar phase then.
- As in the case of the planets (see Study Guide 5), the apparent motions on the sky of the Moon are a composite of its intrinsic motion in its orbit and the motion of our observing platform, the Earth.
- The Moon is the only (large) natural satellite of Earth, moving in a modestly elliptical orbit with an average radius of 238,000 miles (384,000 km). Its sidereal orbital period with respect to the "fixed" stars is 27.3 days. It moves eastward in its orbit as viewed from Earth, about 13 degrees per day (changing rise/set times by about 50 minutes per day). The lunar orbit is tilted slightly (5 degrees) out of the ecliptic plane.
- The situation is shown in the figure above and in this video. In the figure you are looking down on the Earth's North Pole. The Earth spins counterclockwise (in 24 hours), and the Moon orbits counterclockwise (in 29.5 days). The fraction of the Moon's sunlit hemisphere which we can see from Earth determines the lunar phase on a given day.
- We see a "full," "crescent," "gibbous," or dark ("new") Moon depending on the angle between the Sun and Moon as viewed from Earth. The terms "waxing" or "waning" in the diagram refer to whether the visible illuminated portion of the Moon is increasing or decreasing with date.
- The diagram shows that a "first quarter" (or "half-full") Moon is 90o away (east) from the Sun in the sky and that a full Moon is 180o away from the Sun. The Moon will be in a crescent phase when is it less than 90o away from the Sun and a gibbous phase when between 90o and 180o away. The Moon spends about two weeks of each month in each of those conditions.
-
 This
time lapse video composed of still
photographs of the Moon during a 29.5 day cycle vividly illustrates
the relationship between shadowing and phases. The changes in the
apparent size of the Moon and the slight "rocking" motion (known as
libration) are caused by the fact that the lunar orbit is
non-circular in shape.
This
time lapse video composed of still
photographs of the Moon during a 29.5 day cycle vividly illustrates
the relationship between shadowing and phases. The changes in the
apparent size of the Moon and the slight "rocking" motion (known as
libration) are caused by the fact that the lunar orbit is
non-circular in shape.
- Because Earth moves in its own orbit around the Sun, the Moon does not return to the same position with respect to the Sun as seen from Earth (e.g. 180 degrees away from Sun) for 29.5 days, 2.2 days longer than its sidereal period. This is called its synodic period. The situation is shown here.
- The Moon repeats its phases after its synodic period of 29.5 days, which is the basis for our calendrical "month". The phases are almost, but not quite, synchronous with our calendrical months (of 28, 30, or 31 days). The phase of the moon on a given day of the month therefore shifts systematically throughout the year and from one year to the next.
- In a more subtle background cycle, the orientation of the Moon's orbit with respect to the ecliptic changes over an 18.6 year period, which affects the dates of the maximum/minimum excursions of the Moon from the celestial equator and the timing of eclipses (see below).
- You can use the figure above to determine the time of day when the Moon in a given phase will rise, transit, or set. Use the concept of the horizon plane, and note that the marked positions A, B, C, and D correspond to places where observers experience noon, sunset, midnight, and sunrise, respectively.
- For instance, drawing a horizon plane at B (a flat line, just touching the Earth's surface at the observer's position) allows you to infer that a first quarter Moon will transit at sunset; a full Moon rises at sunset; and a waning gibbous Moon is not visible at sunset (because it is below the horizon and not yet risen).
B. Polar Precession
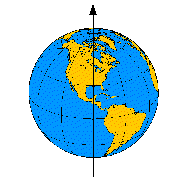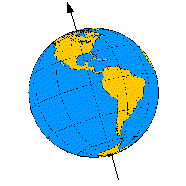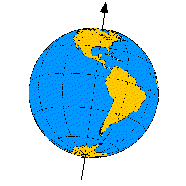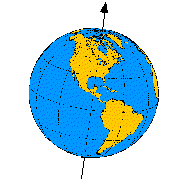
Precession is a motion of the Earth, not the Moon, but it is partly induced by the Moon's motion in its orbit. It is a cyclical, long-period wobble in the orientation of the Earth's polar axis projected on the celestial sphere. This is shown schematically in the animation at the right.- Precession is produced by the combined gravity of the Moon & Sun acting on the "bulge" at Earth's equator. This results in a gradual cyclical change in the direction on the celestial sphere toward which the Earth's poles point. Precessional effects are very slow; the polar cycle lasts 26,000 years, corresponding to an average shift in stellar positions on the sky of only about 0.5 degree per century.
- Though subtle, precession was first detected in 150 BC by
the Greek astronomer Hipparchos through comparison of measured
star positions over several centuries.
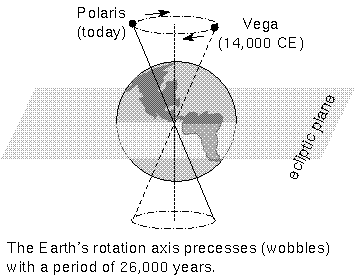
- As shown in the drawing above, Polaris is a
convenient "North Pole Star" now and lies about 1 degree from
the true North Celestial Pole. However, because of precession it will
not be near the pole in a few 1000 years. The bright star Vega will
be close to the pole 12,000 years from now -- but most of the time
there is NO useful pole star. The animation below shows how
the northern constellations change location with respect to the pole
over a period of 11,000 years. (Note: the point labeled "zenith" in
the drawing is actually the "North Pole".)
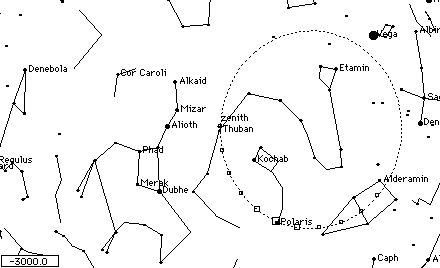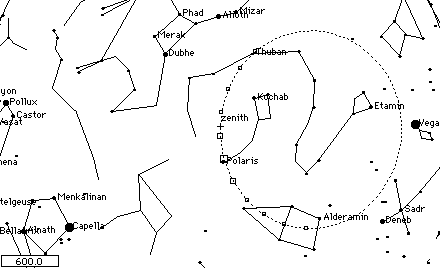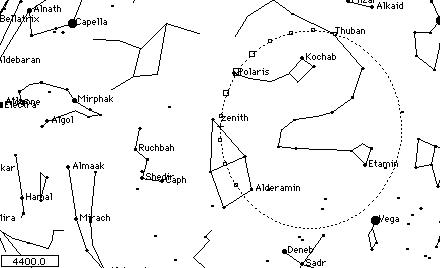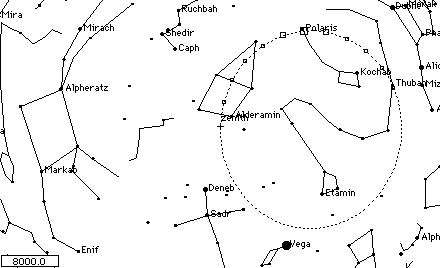
- Precession causes changes in the positions of all stars with respect to the celestial poles and equator. The maximal change in the angle between the pole and a given star is 47o. This means that many stars in the southern hemisphere, which now are always below the horizon from Charlottesville, will become visible at some time in the future, and other familiar stars will shift out of view.
- Precession changes the location of the intersections between the ecliptic and the equator (i.e. the equinoxes) in the stellar reference frame. E.g. the Vernal Equinox moves from one constellation of the Zodiac to the next in about 2150 years.
- Precession is a complication in interpreting ancient astronomical observatories because it causes misalignments between the original building sight lines and the current-day positions of stars. We must take it into account in interpreting ancient structures.
- Technical note: Precession causes the Right Ascension and Declination coordinates of all astronomical objects to continually change slowly. The maximum annual change is about 10 seconds of time in RA and 20 seconds of arc in DEC. All listings of RA,DEC must have the "epoch" -- i.e. the date for which they are valid -- specified. Most listings now give epoch 2000 coordinates.
C. Eclipses (Dark Shadows)
Eclipses are shadow effects in which either the Sun or the Moon appears to "go out" for a time. Both can be beautiful and dramatic events for properly situated observers on Earth. In particular, total solar eclipses have tremendous psychological impact because the Sun seems to disappear in the middle of an ordinary day with no guarantee of return. The sudden extinguishing of the Sun can be terrifying for people who believe the Sun is a living god if they could not predict it in advance. The picture at the top of the page shows a series of photographs taken before, during, and after a total solar eclipse.- There are two types of eclipses: lunar
and solar. They are produced by shadows cast by
the Earth and the Moon, respectively.
- A lunar eclipse occurs when the shadow of the Earth strikes the Moon
- A solar eclipse occurs when the shadow of the Moon strikes the Earth
- The geometry of the two kinds of eclipses is illustrated in
the following diagrams. (Click for enlargements):
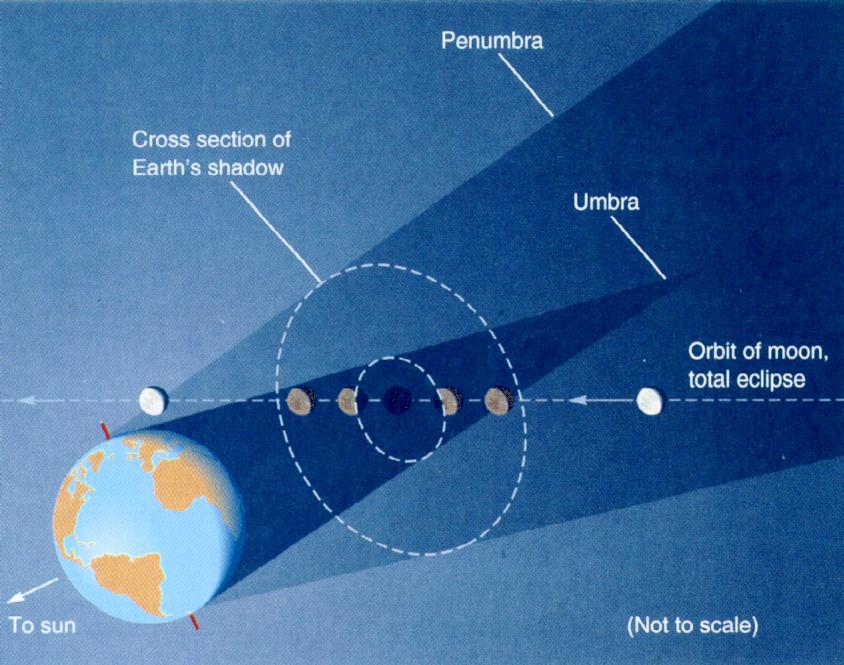
Lunar Eclipse Geometry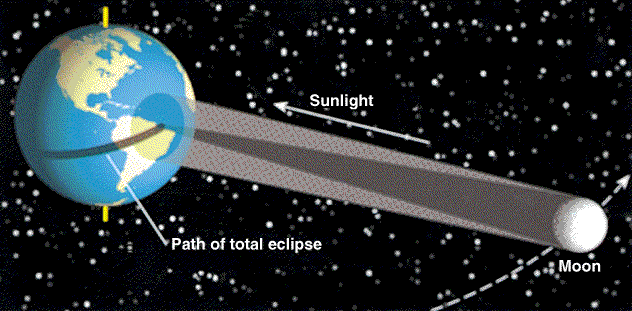
Solar Eclipse Geometry - Referring to these illustrations and the
diagram above
concerning the phases of the Moon, we see that:
- A lunar eclipse can only occur near Full Moon, and
- A solar eclipse can only occur near New Moon
- The core of the shadow, where solar light is totally blocked, is called the umbra. The umbra is shown as the dark cone in the diagrams above. It is surrounded by a larger region (lighter gray above) with partial blocking, called the penumbra. Eclipses can therefore be either "total" or "partial", depending on which part of the shadow is involved.

Two solar eclipses. (Left) Total eclipse in March 1970 from Virginia.
(Right) Annular eclipse in May 2012 from Texas (J. Thumberger).
Solar Eclipses
- As viewed from Earth, the Moon has an angular diameter of about 0.5
degrees. This is almost exactly the same as
the Sun's.
-
This similarity of apparent size is merely a coincidence, a
product of the present-day size of the Moon's orbit (which is
continually changing slowly). The Moon and Sun have, of course,
vastly different intrinsic sizes.
- This coincidence of sizes permits the occurrence of total
solar eclipses on the Earth. In a total eclipse, the umbra of
the Moon's shadow touches the surface of the Earth, as shown in the
Solar Eclipse Geometry diagram above. As seen from locations inside
the umbra, the Moon just barely blocks all of the bright surface of
the Sun. The umbra covers only
a small part
of the Earth's surface at any time, but
 as the Moon moves in its orbit, the
umbra sweeps
across the Earth, producing an elongated strip within which a
total eclipse is experienced.
as the Moon moves in its orbit, the
umbra sweeps
across the Earth, producing an elongated strip within which a
total eclipse is experienced.
- At the right is a time lapse video of a total solar eclipse. The Moon crosses in front of the Sun from right to left. Just as totality is beginning, the exposure time of the camera was increased and shows the "diamond ring" effect produced by the last small uncovered parts of the solar surface.
- For viewers situated in the penumbra, only part of the Sun's surface is blocked by the Moon, producing a partial solar eclipse. Observable effects can be detected without optical aid for eclipse fractions larger than about 5%. "Annular" eclipses are produced when the Moon appears to lie entirely within the Sun's surface; this can occur when the Moon is near its apogee (farthest from Earth in its orbit).
- Partial eclipses are much less dramatic than are total eclipses
because even a small uncovered fraction of the Sun's surface produces
large amounts of light; however, they last longer, are more
frequent, and can be seen from a larger part of the Earth's
surface.

The solar atmosphere during total eclipses.
(Left) Coronal streamers during the February 1998 eclipse (A. Gada, processed by J. Lodriguss).
(Right) Chromosphere showing (red) prominences during August 1999 eclipse (L. Viatour). - During a total solar eclipse, when the tremendously bright surface
of the Sun is just blocked by the Moon, we can observe the very
faint structures surrounding the Sun's surface (called the
"chromosphere" and "corona") that are
normally hidden in the glare.
-
The corona is the irregular white halo surrounding the darkened Sun in
the left hand frame of the picture above. You can also see the inner
corona in the video above (a thin annular glow during totality). The
chromosphere is the region just above the Sun's surface in the right
hand frame of the picture above. The red color of the
flamelike prominences
extending above the solar surface gives the chromosphere
("color"-sphere) its name.
Although special telescope designs can reject much of the light of the
solar surface, total solar eclipses still offer the best views of the
inner solar atmosphere for ground-based telescopes, so they are
very important events scientifically.
- Total solar eclipses last at most 7 minutes at a given location and are visible only in a narrow strip on the Earth's surface. The umbral shadow moves along the strip at a speed of about 1500 mph. Because of the narrow path, relatively few people are able to experience a given total eclipse.
- If the Moon were more distant from Earth, such that the Sun appeared much larger than the Moon, there would never be total solar eclipses. And if the Moon were nearer, so that it appeared much larger than the Sun, total solar eclipses would last longer and be more frequent, though they would be much less interesting aesthetically and scientifically.
- Perhaps the most widely viewed total solar eclipse in history was the "Great American Eclipse" of 21 August 2017, during which the umbra sliced across the United States from the Oregon coast to South Carolina in about 90 minutes. Because of tremendous media coverage, millions of people traveled to the eclipse path. Over 200 million people were estimated to have watched the eclipse, either in total or partial phase. Here is a mosaic of eclipse event pictures.
Lunar Eclipses
- Lunar eclipses can also be total or partial, depending on the exact path of the Moon through the Earth's shadow cones (see this chart of the Moon's track during a typical total eclipse).
- During a total lunar eclipse, the Moon will become very dim. But residual sunlight passing through dust layers in Earth's atmosphere often tints the Moon bloody red, as in the picture below. Click here for an enlarged view of an eclipse.
- By contrast with a total solar eclipse, a total lunar eclipse can last up to 1.5 hours and is visible from about 1/2 of the Earth's surface. Most of Earth's inhabitants (with astronomical interests) have therefore seen lunar eclipses.

Multiple exposure of a 2008 lunar eclipse over the Southern Cascade Mountains.
The exposure time was increased during the faintest phases. (Sean Bagshaw)
D. Eclipse Prediction
The basic geometry of eclipses is simple, but predicting their occurrence and type (total, partial, annular) depends on understanding the complex nature of the lunar orbit:- Almost perfect alignment of the Sun, the Moon and the Earth is needed for an eclipse.
- The Earth and Sun lie (by definition) in the ecliptic plane and therefore the Moon must also be in that plane for an eclipse to occur. (Hence, the origin of the word "ecliptic.")
- If the Moon's orbit lay exactly in the plane of the ecliptic and were perfectly circular, there would be a total eclipse of the Sun and a total eclipse of the Moon at each new and full Moon, respectively, each month. Eclipses would be routine celestial phenomena, and the psychological impact of solar eclipses would greatly diminish.
- But the Moon's orbit is tilted 5 degrees out of the ecliptic
plane and only crosses the plane at two points, called
the nodes. The Moon moves through the two nodes once
each month.
-
5 degrees sounds small, but remember that it is 10 times the angular
diameter of the Moon.
- Therefore, the Moon must be near one of its
nodes and that node must lie almost directly on the line
running from the Sun through the Earth for eclipses to occur.
- Viewed on the celestial sphere from the Earth, the node is where the
Moon's celestial path crosses the ecliptic. See the diagram below
(click for enlargement). Only if the Sun and Moon are both near the
node at the same time can a solar eclipse occur. If the Sun and Moon
are both close to the node but the alignment is not perfect, a
partial eclipse will occur, as in the figure.
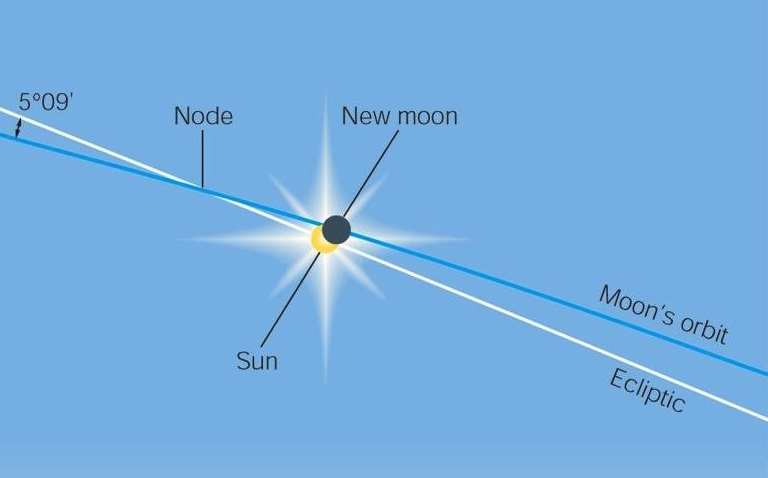
- During the few days immediately before and after a solar eclipse the Moon (a thin crescent) is very hard to see in the sky. Cultures without a practical understanding of astronomy could be taken unawares by a solar eclipse and fall into panic at the darkening of the Sun.
- Viewed on the celestial sphere from the Earth, the node is where the
Moon's celestial path crosses the ecliptic. See the diagram below
(click for enlargement). Only if the Sun and Moon are both near the
node at the same time can a solar eclipse occur. If the Sun and Moon
are both close to the node but the alignment is not perfect, a
partial eclipse will occur, as in the figure.
- The direction to a node on the celestial sphere (i.e. with respect to the stars) changes, but only slowly. Good alignments between the "line of nodes" and the Sun-Earth line therefore only occur at approximately 6-month intervals. [Equivalently, once the Sun has passed one node along its ecliptic path, it will not approach the other for 6 months.]
- The perspective drawing below shows the
orientation of the Moon's orbit in 3-D space. Eclipses occur only near
those times when the line of nodes points near the Sun (called
"eclipse seasons" and marked "favorable for eclipse" in the
drawing). On average, there are 2 solar and 2 lunar eclipses each
year.
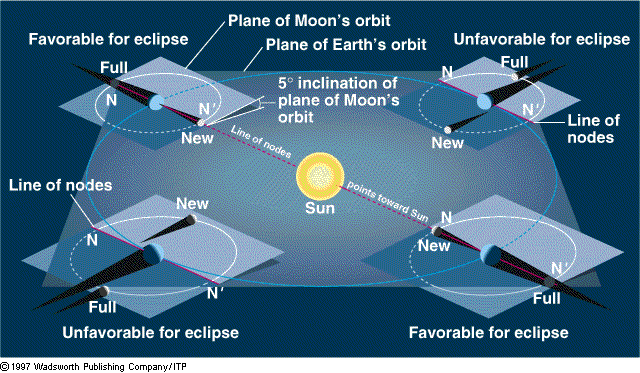
- Because of gravitational interactions between the Moon and Sun,
the line of nodes of the Moon's orbit moves with time,
taking 18.6 years to make a complete rotation around the Earth.
This produces a 18.6-year-long sequence of eclipses called
the saros cycle.
-
During the cycle, solar and lunar eclipses occur roughly every 6
months. But the pattern of months in which eclipses occur change and
don't repeat for about 19 years.
The dates and paths on the Earth's surface for solar eclipses
from 2000 to 2020 are shown here.
- The change in the line of nodes also produces changes in the extreme northerly or southerly positions of the Moon as seen from Earth in a given month by +/- 5 degrees. This affects, for instance, the duration of a moonlit night, so that the cycle can be recognized without necessarily observing eclipses. The saros cycle was known to many ancient astronomers, including those who helped build Stonehenge (see Study Guide 5).
- More variety is thrown into the character of eclipses by the fact that the Moon's orbit is elliptical in shape, meaning that the Moon can be at different distances from the Earth during eclipse seasons; when it is farther from Earth, an annular rather than a total eclipse can occur.
Web links:
-
Lunar
Phase Now
Espenak's Eclipse Home Page: everything you could possibly want to
know about eclipses.
The USA Total Solar Eclipse of 21 August
2017 (NASA)
 Back to Study Guide 4
Back to Study Guide 4
|
 Guide Index
Guide Index
|